3 Optimal spike
generation frequency
The spike in the neuron is a probabilistic event:
excitability of neuron l 0=1/P0 corresponds to the probability of a single spike
appearance per unit of time. Besides q,
excitability l 0
depends on other factors, and they together may cause l 0>>1, that turns
neuron into the threshold element. Conditions of generation can be
conveniently considered on the plot of dependence of the neuron's
dynamic threshold Pd on time t
, passed from the last spike instant. Dependence Pd(t ) is well known in neurophysiology and is shown
in fig.2.
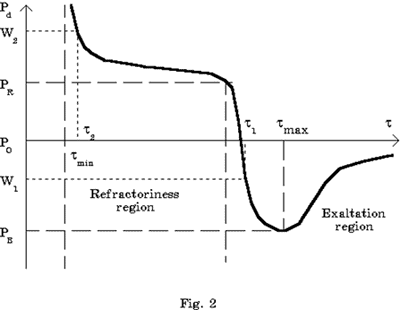
Pd varies in broad limits on a very
small time interval, during which the values of other factors,
influencing the neuron's excitability, practically do not vary.
Therefore, the second and succeeding spikes in the series of spikes may
be considered as threshold events. The spike takes place at the
fulfillment of condition
W ³ P0+Ps+Pd ,
where W is the excitation potential, that is the result of
the spatio-temporal summation of influences on the neuron from
excitatory and inhibitory links, and Ps is the
static threshold, which accounts for the influence of preceding spikes.
If potential W is equal to small value W1, then
after the first random spike the neuron will begin to generate spikes
with the time interval t 1,
i.e., will generate at low frequency (LF) 1/t
1 in the exaltation phase. At the great
potential value W2 the neuron will generate at
high frequency (HF) 1/t 2
in the phase of deep refractoriness. Since every spike decreases
neuron's age q , the variation of t will vary an energetic cost for decrease of q . We have shown earlier, that there is a
certain optimal time topt,
which provides a minimal energetic cost. Parameters of the model can be
selected by such a way, that topt
would situate in the region of deep refractoriness; this creates
conditions for the self-organization of the automaton: the most natural
and easy generation mode in the exaltation phase is energetically
unprofitable, while the neuron cannot itself reach the frequency of
generation uopt=1/topt and therefore must
interact with other neurons. The tendency to generate at optimal
frequency is provided by the optimal learning rule [2]. The key idea of this rule is expressed
by formula
D r i j=ui(uopt-uj),
where r i j
is the conductivity of an excitatory link from i-th to j-th neuron.
It is important for the completeness of the concept, that
besides generation at uopt
the neuron has another energetically profitable working mode: random
spikes with intervals, much greater than time te, which corresponds to the minimum of Pd.